How, On Earth, Do We Measure the Universe?

Measurements using similar triangles.

images and article by Steven Ransom-Jones

One of my fondest childhood memories, inspired by a great teacher who saw my interest in physics, revolves around borrowing the school telescope over the Christmas holidays. For a few cool, clear winter nights I was able to share with my family some of the wonders of the sky. This was the first time that we had seen, first hand, details of lunar craters, the rings of Saturn, the Andromeda Galaxy as a disk as well as Jupiter’s moons and stripes. With the enthusiasm of a 12-year-old, I was able to rattle off facts about the distances and sizes of these objects.

My mother brought me “down to earth” with the question, but how do we know that these distances are true if we have never been there?

After getting over this blow to my faith in textbooks, a healthy curiosity was inspired as well as a realization that we have learned so much about distant space before we had ever ventured from the Earth. I became determined to understand how, on earth, we had measured so much about the Universe.

The tools needed to start on this journey are quite basic. With a method to measure time and space (a ruler and a watch will suffice for now) and armed with a basic understanding of angles and triangles, we are ready to begin.

A celestial triangle to measure the distance of the sun.

Let us start with the Earth. If we went to Syene, a city near the equator, around 2,200 years ago, we may have met Eratosthenes. According to legend, Eratosthenes noticed that at noon on the longest day of the year, he could see the water at the bottom of a very deep well. He deduced that the sun must be directly overhead on this day and time. At the same moment, it was observed that the Column at Alexandria (around 500 miles away) cast a shadow. Knowing the height of the Column and the size of the shadow, he could (using the magic of triangles) determine the angle of the sun from overhead. By multiplying this angle up to 360 degrees and the distance from Syene to Alexandria by the same value he calculated that the Earth was 25,000 miles in diameter (amazingly, within one percent of true value).

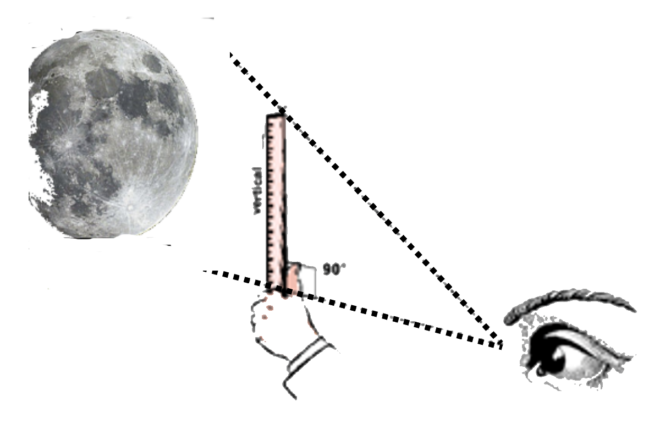
Turning our eyes to our nearest neighbor, the moon, using a simple “similar triangle” apparatus then, we know the angle that the moon represents in the sky. We just need to know either the distance to the moon or the size of the moon to calculate the other dimension.

Aristarchus of Samos, who lived around the same time as Eratosthenes, observed a lunar eclipse, where the moon passes through the shadow cast by the Earth. He timed how long it took for the moon to pass into the Earth’s shadow (around an hour) and then pass completely through it (around two hours and 36 minutes). His deduction was that the Earth’s diameter was approximately 2.6 times that of the moon. His estimate was certainly of the right order of magnitude. Unfortunately the Earth does not project a fully cylindrical shadow out to the moon’s orbit, but there is an area of partial shadow (known as a penumbra). This is due to the relative size and placement of the sun and Earth. Once this was taken into account then the relative diameter of the Earth was refined to 3.7 times that of the moon. We can now use the magic of similar triangles to estimate the distance of the moon.

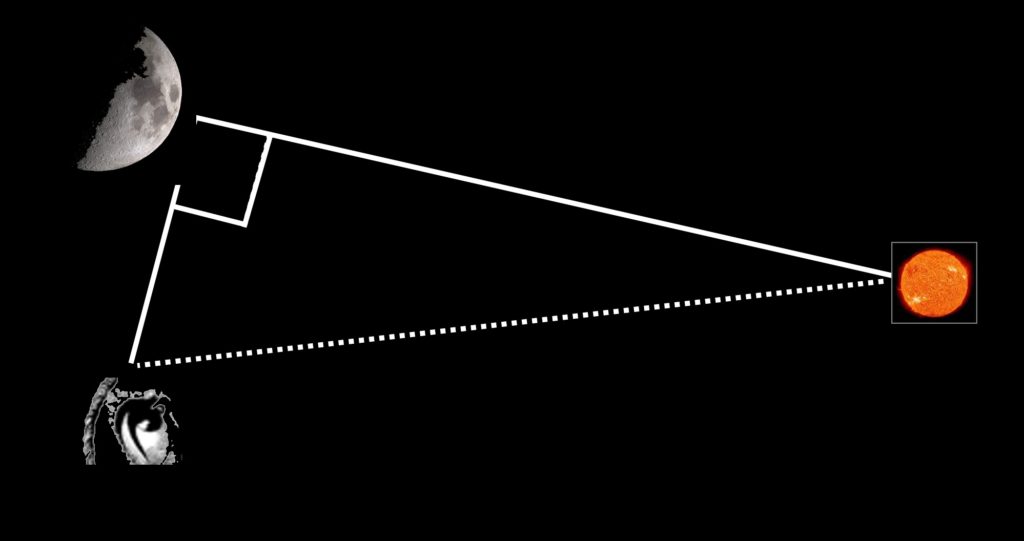
Aristarchus then turned his attention to measuring the distance of the sun. The puzzle was to form a convenient triangle to solve this problem. He applied some deductive reasoning to an observation of the moon: when the moon is half illuminated, then the sun must be directly overhead the moon. The rays of light from the sun, reflecting into our eyes from the half illuminated moon must therefore form a right angle. Aristarchus had created an imaginary right-angled triangle between the sun, moon and observer. If we can just measure the angle of the moon from the vertical then we could deduce the moon to sun distance. Improvements of Aristarchus’ first rough measurement eventually placed the sun at 390 lunar distances, or 93 million miles.

The challenge for measuring astronomical distances is rapidly becoming one of measuring angles as precisely as possible.

Using parallax to measure distances.

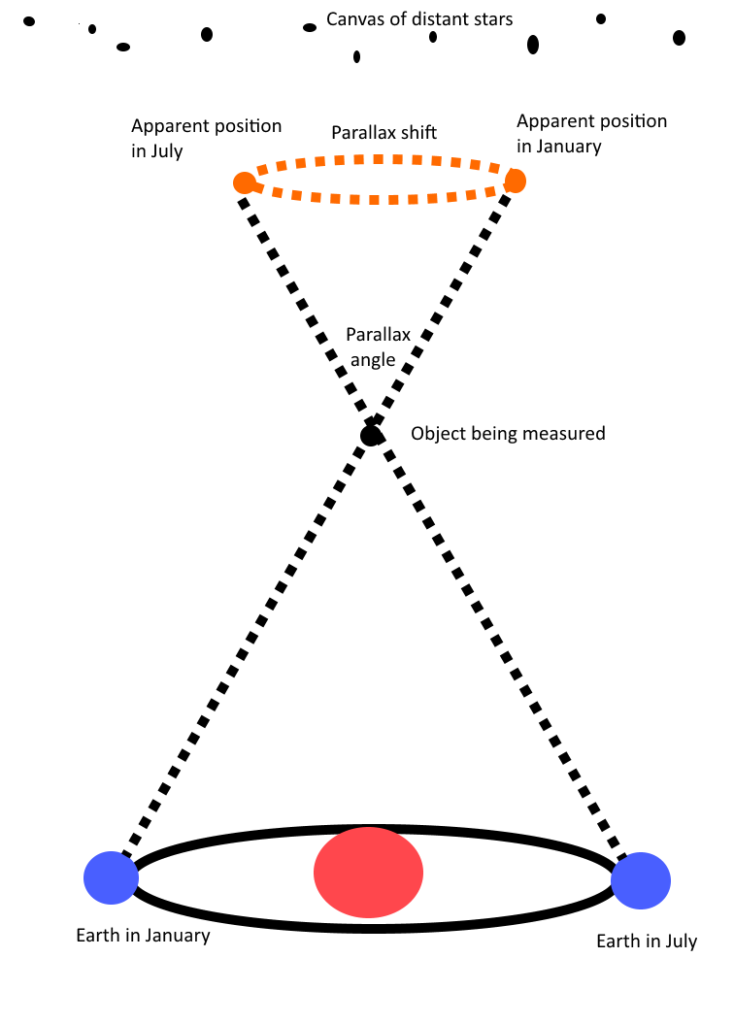
One severe limitation when measuring vast distances using simple instruments is that it is easy to lose precision. Just a small measurement error makes a significant difference to the result. We need to make the “instrument” triangles as large as possible to obtain the maximum precision. To obtain better distance measurements (for either more accuracy or to measure greater distances), we need to use triangles with the largest possible base side. Astronomers were able to construct these large triangles by simultaneously making observations of the sun, moon or planets from different places on the Earth (using our whole planet as one side of the triangle). Small angles could be measured by looking at the apparent differences in the position of nearby objects against the backdrop of distant stars (known as the parallax effect). Now we can estimate the distance of the planets.

By making observations six months apart (when the Earth is on opposite sides of the sun), the base of the triangle is extended to 186 million miles. This allowed more accurate measurements of the distances of the planets and even the ability to determine the proximity of our nearest stellar neighbors.

With some simple tools and creative thinking, centuries before we could fly even a few hundred feet above the ground, mankind had started to reach for, and understand, the celestial objects around us. While I end this article with the nearest stars in our conceptual grasp, it is possible to continue this exploration across the Milky Way, to other galaxies and possibly to the edges of the universe from the comfort of our back yards.

“Eye On the Night Sky” is a monthly column by the Door Peninsula Astronomical Society. The Door Peninsula Astronomical Society meets on July 11 at 7 pm at the Stonecipher Astronomy Center, 2200 Utah St. in Sturgeon Bay. The topic will be the 2017 solar eclipse and tips on how to safely view it. For more information on the organization, visit DoorAstronomy.org.